Chapter 14 Quadrilaterals R.D. Sharma Solutions for Class 9th MCQ's
Multiple Choice Questions
1. The opposite sides of a quadrilateral have
(a) no common point
(b) one common point
(c) two common points
(d) infinitely many common points
Solution

2. The consecutive sides of a quadrilateral have
(a) no common point
(b) one common point
(c) two common points
(d) infinitely many common points
Solution
3. PQRS is a quadrilateral, PR and QS intersect each other at O. In which of the following cases, PQRS is a parallelogram?
(a) ∠P = 100°, ∠Q = 80°, ∠R = 95°
(b) ∠P =85°, ∠Q = 85°, ∠R = 95°
(c) PQ = 7 cm, QR = 7 cm, RS = 8 cm, SP = 8 cm
(d) OP = 6.5 cm, OQ = 6.5 cm, OR = 5.2 cm, OS = 5.2 cm
Solution
Let us analyze each case one by one.
We have a quadrilateral named PQRS, with diagonals PR and QS intersecting at O.
(a) ∠P=100°, ∠Q = 80°, ∠R=100°
By angle sum property of a quadrilateral, we get:
∠P + ∠Q + ∠R + ∠S = 360°
⇒ 100° +80° +100° + ∠S = 360°
⇒ 280° + ∠S = 360°
⇒ ∠S - 80°
Clearly, ∠P = ∠R
And, ∠Q = ∠S
Thus we have PQRS a quadrilateral with opposite angles are equal.
Therefore, PORS is a parallelogram.
(b) ∠P = 85°, ∠Q=85°, ∠R=95°
By angle sum property of a quadrilateral, we get:
∠P + ∠Q + ∠R + ∠S = 360°
⇒ 85° +85° +95° + ∠S = 360°
⇒ 265° + ∠S = 360°
∠S = 95°
Clearly, ∠P ≠ ∠R
And ∠Q ≠ ∠S
Thus we have PQRS a quadrilateral with opposite angles are not equal.
Therefore, PORS is not a parallelogram.
(c) PQ = 7cm, QR = 7cm, RS = 8cm, SP = 8cm
Clearly, PQ ≠ RS
And QR ≠ SP
Thus, we have PQRS a quadrilateral with opposite sides are not equal.
Therefore,
PQRS is not a parallelogram.
(d) OP = 6.5cm, OQ = 6.5cm, OR = 5.2cm, OS = 5.2cm
We know that the diagonals of a parallelogram bisect each other.
But, here we have
OP ≠ OR
And OQ ≠ OS
Therefore, PQRS is not a parallelogram.
Hence, the correct choice is (a).
4. Which of the following quadrilateral is not a rhombus?
(a) All four sides are equal
(b) Diagonals bisect each other
(c) Diagonals bisect opposite angles
(d) One angle between the diagonals is 60°
Solution

5. Diagonals necessarily bisect opposite angles in a
(a) rectangle
(b) parallelogram
(c) isosceles trapezium
(d) square
Solution

6. The two diagonals are equal in a
(a) parallelogram
(b) rhombus
(c) rectangle
(d) trapezium
Solution

7. We get a rhombus by joining the mid-points of the sides of a
(a) parallelogram
(b) rhombus
(c) rectangle
(d) triangle
Solution

8. The bisectors of any two adjacent angles of a parallelogram intersect at
(a) 30°
(b) 45°
(c) 60°
(d) 90°
Solution

9. The bisectors of the angle of a parallelogram enclose a
(a) parallelogram
(b) rhombus
(c) rectangle
(d) square
Solution

10. The figure formed by joining the mid-points of the adjacent sides of a quadrilateral is a
(a) parallelogram
(b) rectangle
(c) square
(d) rhombus
Solution

11. The figure formed by joining the mid-points of the adjacent sides of a rectangle is a
(a) square
(b) rhombus
(c) trapezium
(d) none of these
Solution

12. The figure formed by joining the mid-points of the adjacent sides of a rhombus is a
(a) square
(b) rectangle
(c) trapezium
(d) none of these
Solution

13.The figure formed by joining the mid-points of the adjacent sides of a square is a
(a) rhombus
(b) square
(c) rectangle
(d) parallelogram
Solution

14. The figure formed by joining the mid-points of the adjacent sides of a parallelogram is a
(a) rectangle
(b) parallelogram
(c) rhombus
(d) square
Solution

15. If one angle of a parallelogram is 24° less than twice the smallest angle, then the measure of the largest angle of the parallelogram is
(a) 176°
(b) 68°
(c) 112°
(d) 102°
Solution

16. In a parallelogram ABCD, if ∠DAB = 75° and ∠DBC = 60°, then ∠BDC =
(a) 75°
(b) 60°
(c) 45°
(d) 55°
Solution

17. ABCD is a parallelogram and E and F are the centroids of triangles ABD and BCD respectively, then EF =
(a) AE
(b) BE
(c) CE
(d) DE
Solution

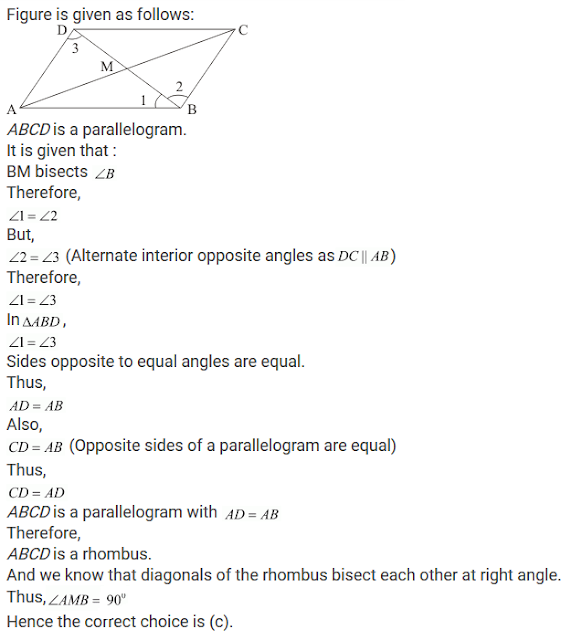
18. ABCD is a parallelogram, M is the mid-point of BD and BM bisects ∠B. Then ∠AMB =
(a) 45°
(b) 60°
(c) 90°
(d) 75°
Solution
19. ABCD is a parallelogram and E is the mid point of BC. DE and AB when produced meet at F. Then AF
(a) 3/2 AB
(b) 2 AB
(c) 3 AB
(d) 5/4 AB

20. If an angle of a parallelogram is two-third of its adjacent angle, the smallest angle of the parallelogram is
(a) 108°
(b) 54°
(c) 72°
(d) 81°
Solution

21. If the degree measures of the angles of quadrilateral are 4x, 7x, 9x and 10x, what is the sum of the measures of the smallest angle and largest angle?
(a) 140°
(b) 150°
(c) 168°
(d) 180°
Solution

22. In a quadrilateral ABCD, ∠A + ∠C is 2 times ∠B + ∠D. If ∠A = 140° and ∠D = 60°, then ∠B =
(a) 60°
(b) 80°
(c) 120°
(d) None of these
Solution

23. If the diagonals of a rhombus are 18 cm and 24 cm respectively, then its side is equal to
(a) 16 cm
(b) 15 cm
(c) 20 cm
(d) 17 cm
(a) 16 cm
(b) 15 cm
(c) 20 cm
(d) 17 cm
Solution

(a) 70°
(b) 90°
(c) 80°
(d) 100°
Solution

(a) 70°
(b) 110°
(c) 90°
(d) 120°
Solution

26. In a rhombus ABCD, if ∠ACB = 40°, then ∠ADB =
(a) 70°
(b) 45°
(c) 50°
(d) 60°
Solution

27. In ΔABC, ∠A = 30°, ∠B = 40° and ∠C = 110°. The angles of the triangle formed by joining the mid-points of the sides of this triangle are
(a) 70°, 70°, 40°
(b) 60°, 40°, 80°
(c) 30°, 40°, 110°
(d) 60°, 70°, 50°
(a) 70°, 70°, 40°
(b) 60°, 40°, 80°
(c) 30°, 40°, 110°
(d) 60°, 70°, 50°
Solution
28.The diagonals of a parallelogram ABCD intersect at O. If ∠BOC = 90° and ∠BDC = 50°, then ∠OAB =
(a) 40°
(b) 50°
(c) 10°
(d) 90°
(a) 40°
(b) 50°
(c) 10°
(d) 90°
Solution

(a) 10 cm
(b) 12 cm
(c) 14 cm
(d) 16 cm
Solution

30. Diagonals of a quadrilateral ABCD bisect each other. If ∠A= 45°, then ∠B =
(i) 115°
(ii) 120°
(iii) 125°
(iv) 135°
Solution

31. P is the mid-point of side BC of a parallelogram ABCD such that ∠BAP = ∠DAP. If AD = 10 cm, then CD =
(a) 5 cm
(b) 6 cm
(c) 8 cm
(d) 10 cm
Solution

32. In ΔABC, E is the mid-point of median AD such that BE produced meets AC at F. IF AC = 10.5 cm, then AF =
(a) 3 cm
(b) 3.5 cm
(c) 2.5 cm
(d) 5 cm
Solution



