Chapter 3 Pair of Linear Equations in Two Variables R.D. Sharma Solutions for Class 10th Math Exercise 3.4
Exercise 3.4
1. Solve each of the following systems of equations by the method of cross-multiplication:-
1. x + 2y + 1 = 0
2x - 3y - 12 = 0
Solution
The given system of equation is
Hence, the solution of the given system of equations is x = 3, y = -2.
2. 3x + 2y + 25 = 0
2x + y + 10 = 0
Solution
The given system of equation is
Hence, x = 5, y = -20 is the solution of the given system of equations.
3. 2x + y - 35 = 0
3x + 4y - 65 = 0
Solution
The given system of equations may be written as
Hence, x = 15, y = 5 is the solution of the given system of equations.
4. 2x - y - 6 = 0
x - y - 2 = 0
Solution
The given system of equations may be written as
Hence , x = 4, y = 2 is the solution of the given system of the equations.
5. (x+y)/xy = 2
(x-y)/xy = 6
Solution
The given system of equations is
6. ax + by = a - b
bx - ay = a + b
Solution
The given system of equations is
Hence, x = 1, y = 1 is the solution of the given system of the equations.
7. x + ay - b = 0
ax - by - c = 0
Solution
The given system of equations may be written as
8. ax + by = a2
bx + ay = b2
Solution
The system of the given equations may be written as
Solution
The given system of equation is
11. 57/(x+y) + 6/(x-y) = 5
38/(x+y) + 21/(x-y) = 9
Hence we get the value of x = 11 and y = 8 .
Solution
Solution
15. 2ax + 3by = a + 2b
3ax + 2by = 2a + b
Solution
The given system of equations is
16. 5ax + 6by = 28
3ax + 4by - 18 = 0
Solution
The given system of equation is
17. (a+2b)x + (2a-b)y = 2
(a-2b)x + (2a+b)y = 3
Solution
The given system of equations may be written as
Solution
The given system of equation is
19. bx + cy = a + b
ax[1/(a-b) - 1/(a+b)] + cy[1/(b-a) - 1/(b+a) = 2a/(a+b)
Solution
The given system of equation is
20. (a-b)x + (a+b)y = 2a2 - 2a2
Solution
The given system of equation is
21. a2x + a2y = c2
Solution
The given system of equations may be written as
Solution
23. 2(ax-by) + a + 4b = 0
2(bx+ay) + b - 4a = 0
Solution
The given system of equation may be written as
Solution
Solution
26. mx - my = m2 + n2
x + y = 2m
Solution
The given system of equations may be written as
Solution
The given system of equation may be written as
28. bx/a + ay/b - (a2 + b2) = 0
x + y - 2ab = 0
Solution
The given system of equation may be written as
1. x + 2y + 1 = 0
2x - 3y - 12 = 0
Solution
The given system of equation is
2. 3x + 2y + 25 = 0
2x + y + 10 = 0
Solution
The given system of equation is
3. 2x + y - 35 = 0
3x + 4y - 65 = 0
Solution
The given system of equations may be written as
4. 2x - y - 6 = 0
x - y - 2 = 0
Solution
The given system of equations may be written as
5. (x+y)/xy = 2
(x-y)/xy = 6
The given system of equations is
6. ax + by = a - b
bx - ay = a + b
Solution
The given system of equations is
7. x + ay - b = 0
ax - by - c = 0
Solution
The given system of equations may be written as
bx + ay = b2
Solution
The system of the given equations may be written as
9. 5/(x+y) - 2/(x-y) = -1
15/(x+y) + 7/(x-y) = 10
where x ≠ 0 and y ≠ 0
Solution
10. 2/x + 3/y = 13
5/x - 4/y = -2
where x ≠ 0 and y ≠ 0
The given system of equation is
11. 57/(x+y) + 6/(x-y) = 5
38/(x+y) + 21/(x-y) = 9
Solution
Now adding eq.(3) and (4) we get x = 11
And after substituting the value of x in eq. (4) we get y = 8Hence we get the value of x = 11 and y = 8 .
12. x/a + y/b = 2
ax-by = a2 - b2
Solution
The system of the given equations may be written as .
13. x/a + y/b = a+b
x/a2 + y/b2 = 2
Hence we get the value = a2 and y = b2
14. x/a = y/b
ax + by = a2 + b2
15. 2ax + 3by = a + 2b
3ax + 2by = 2a + b
Solution
The given system of equations is
16. 5ax + 6by = 28
3ax + 4by - 18 = 0
Solution
The given system of equation is
17. (a+2b)x + (2a-b)y = 2
(a-2b)x + (2a+b)y = 3
Solution
The given system of equations may be written as
18. x[a-b + ab/(a-b)] = y[a+b - ab/(a+b)]
x+y = 2a2
The given system of equation is
19. bx + cy = a + b
ax[1/(a-b) - 1/(a+b)] + cy[1/(b-a) - 1/(b+a) = 2a/(a+b)
Solution
The given system of equation is
20. (a-b)x + (a+b)y = 2a2 - 2a2
(a+b) (x+y) = 4ab
Solution
The given system of equation is
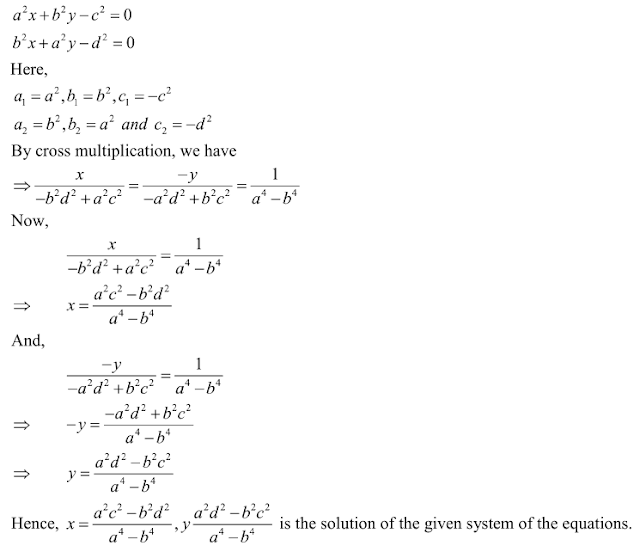
21. a2x + a2y = c2
b2x + a2y = d2
Solution
The given system of equations may be written as
22. 57/(x+y) + 6/(x-y) = 5
38/(x+y) + 21/(x-y) = 9
23. 2(ax-by) + a + 4b = 0
2(bx+ay) + b - 4a = 0
Solution
The given system of equation may be written as
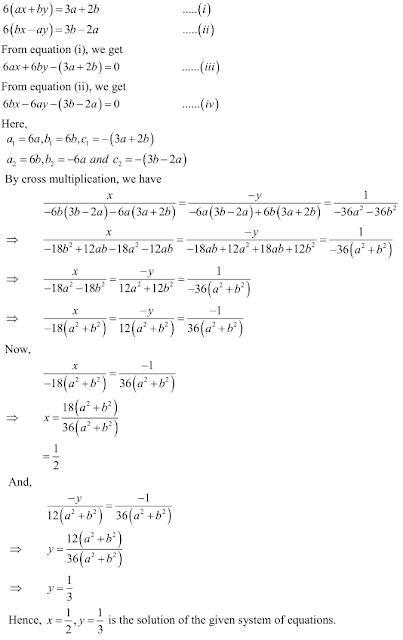
24. 6(ax + by) = 3a + 2b
6(bx - ay) = 3b - 2a
Solution
25. a2/x - b2/y = 0
a2b/x + c2a/y = a + b, x,y ≠ 0
26. mx - my = m2 + n2
x + y = 2m
Solution
The given system of equations may be written as
27. ax/b - by/a = a+b
ax - by = 2ab
Solution
The given system of equation may be written as
28. bx/a + ay/b - (a2 + b2) = 0
x + y - 2ab = 0
The given system of equation may be written as