Chapter 2 Polynomials Important Questions for CBSE Class 10 Maths Board Exams
Important Questions for Chapter 2 Polynomials Class 10 Maths
Polynomials Class 10 Maths Important Questions Very Short Answer (1 Mark)
Solution
We know that,

2. If -1 is a zero of the polynomial f(x) = x2 - 7x - 8, then calculate the other zero.
Solution
We have f(x) = x2 - 7x - 8
Let other zero be k, then we have
⇒ k = 8
3. If the sum of zeroes of the quadratic polynomial 3x2 – kx + 6 is 3, then find the value of k.
Solution
Here a = 3, b = -k, c = 6
Sum of the zeroes, (α + β) = −ba = 3 (given)
⇒ −(−k)3 = 3
⇒ k = 9
4. A quadratic polynomial, whose zeroes are -4 and -5, is ….
Solution
x2 + 9x + 20 is the required polynomial.
5. Polynomials of degrees 1, 2 and 3 are called ........, ....... and ........ polynomials respectively.
Solution
linear, quadratic, cubic
6. The algebraic expression in which the variable has non-negative integral exponents only is called ..........
Solution
Polynomial
7. If α and β are the zeroes of a polynomial such that α + β = -6 and αβ = 5, then find the polynomial.
Solution
Quadratic polynomial is x2 – Sx + P = 0
⇒ x2 – (-6)x + 5 = 0
⇒ x2 + 6x + 5 = 0
Polynomials Class 10 Maths Important Questions Short Answer-I (2 Marks)
8. Find all the zeroes of f(x) = x2 - 2x
Solution
f(x) = x2 - 2x
= x(x-2)
Substituting f(x) = 0, and solving we get, x= 0, 2x
Hence, zeroes are 0 and 2.
9. Find the condition that zeroes of polynomial p(x) = ax2 + bx + c are reciprocal of each other.
Solution
Let α and 1/α be the zeroes of f(x).
f(a) = ax2 + bx + c …(given)
Product of zeroes = ca
⇒ α × 1/α = ca
⇒ 1 = ca
⇒ a = c (Required condition)
Coefficient of x2 = Constant term
10. Find the zeroes of the quadratic polynomial √3x2 - 8x + 4√3
Solution
p(x) = √3 x2 - 8x + 4√3
= √3 x2 - 6x – 2x + 4√3
= √3x(x - 2√3) - 2(x - 2√3)
(√3 x -2) (x - 2√3)
Substituting p(x) = 0, we have
(√3 x -2) (x - 2√3)
11. Form a quadratic polynomial p(x) with 3 and -2/5 as sum and product of its zeroes, respectively.
Solution
Sum of zeroes, α+β = 3
Product of zeroes αβ = -2/5
Now,
p(x) = x2 - (α+β)x + αβ

Solution
Quadratic polynomial is
x2 – (Sum of zeroes) x + (Product of zeroes)
= x2 – (0)x + (-√2)
= x2 – √2
13. If the zeroes of the polynomial x2 + px + q are double in value to the zeroes of 2x2 – 5x – 3, find the value of p and q.
Solution
We have, 2x2 – 5x – 3 = 0
= 2x2 – 6x + x – 3
= 2x(x – 3) + 1(x – 3)
= (x – 3) (2x + 1)
Zeroes are:
x – 3 = 0 or 2x + 1 = 0
⇒ x = 3 or x = −12
Since, the zeroes of required polynomial is double of given polynomial.
Zeroes of the required polynomial are:
3×2, (−12×2) i.e. 6, -1
Sum of zeroes = 6 + (-1) = 5
Product of zeroes = 6 × (-1) = -6
Quadratic polynomial is x2 – Sx + P
⇒ x2 – 5x – 6 …(i)
Comparing (i) with x2 + px + q
p = -5, q = -6
14. If m and n are the zeroes of the polynomial 3x2 + 11x - 4, find the value of m/n + n/m.
Solution


Solution
p(x) = 2x2 – x – 6 [Given]
= 2x2 – 4x + 3x – 6
= 2x (x – 2) + 3 (x – 2)
= (x – 2) (2x + 3)
Zeroes are:
x – 2 = 0 or 2x + 3 = 0
x = 2 or x = −32
Verification:
Here a = 2, b = -1, c = -6
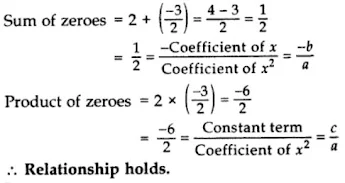
Solution
Let, the zero of 2x2 + 3x + λ be 1/2 and β.
Thus, the other zero is -2.

Solution

(2x – 3) should be subtracted from x4 + 2x3 – 13x2 – 12x + 21.
Polynomials Class 10 Maths Important Questions Short Answer-II (3 Marks)
20. Find a quadratic polynomial whose zeroes are reciprocals of the zeroes of the polynomial f(x) = ax2 + bx + c, a≠0, c≠0.
Solution
Let α and β be zeros of the given polynomial ax2 + bx + c.
α+β = -b/a and αβ = c/a
Let 1/α and 1/β be the zeros of new polynomial then we have


22. Show that 12 and −32 are the zeroes of the polynomial 4x2 + 4x – 3 and verify the relationship between zeroes and co-efficients of polynomial.

Solution
Let Sum of zeroes (α + β) = S = -8 [Given]
Product of zeroes (αβ) = P = 12 [Given]
Quadratic polynomial is x2 – Sx + P
= x2 – (-8)x + 12
= x2 + 8x + 12
= x2 + 6x + 2x + 12
= x(x + 6) + 2(x + 6)
= (x + 2)(x + 6)
Zeroes are:
x + 2 = 0 or x + 6 = 0
x = -2 or x = -6
24. Find the zeroes of the quadratic polynomial 5x2 + 8x - 4 and verify the relationship between the zeroes and the coefficients of the polynomial.
Solution
We have,
p(x) = 5x2 + 8x - 4 = 0
= 5x2 + 10x -2x - 4 = 0
= 5x(x+2) -2(x+2) = 0
= (x+2) (5x-2)
Substituting p(x) = 0 we get zeroes as 2 - and 2/5.
Verification :

Solution
Let P(x) = x3 – 8x2 + 19x – 12
Put x = 1
P(1) = (1)3 – 8(1)2 + 19(1) – 12
= 1 – 8 + 19 – 12
= 20 – 20
= 0
Remainder = 0
(x – 1) is a factor of P(x).
Verification:

Since remainder = 0
(x – 1) is a factor of P(x).
26. When p(x) = x2 + 7x + 9 is divisible by g(x), we get (x+2) and -1 as the quotient and remainder respectively, find g(x).
Solution
We have,
p(x) = x2 + 7x + 9
q(x) = x+2
r(x) = -1
Now,
p(x) = g(x) q(x) + r(x)
x2 + 7x + 9 = g(x) (x+2) -1

Thus, g(x) = x+5
27. Find the value for k for which x4 + 10x3 + 25x2 + 15x + k is exactly divisible by x+7.
Solution
We have,
f(x) = x4 + 10x3 + 25x2 + 15x + k
If x+7 is a factor then -7 is a zero of f(x) and x = -7 satisfy f(x) = 0.
Thus substituting x = -7 in f(x) and equating to zero we have,
(-7)4 + 10(-7)3 + 25(-7)2 + 15(-7) + k = 0
⇒ 2401 - 3430 + 1225 - 105 + k = 0
⇒ 3626 - 3535 + k = 0
⇒ 91 + k = 0
⇒ k = -91
28. If the squared difference of the zeroes of the quadratic polynomial f(x) = x2 + px + 45 is equal to 144, find the value of p.
Solution
We have,
f(x) = x2 + px + 45
Let α and β be the zeroes of the given quadratic polynomial.
Sum of zeroes, α+β = −p
Product of zeroes, αβ = 45
Given,
(α-β)2 = 144
⇒ (α+β)2 + 4αβ = 144
Substituting value of α+β and αβ we get
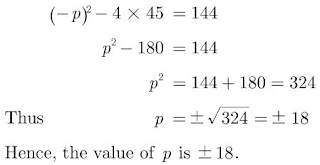
Polynomials Class 10 Maths Important Questions Long Answer (4 Marks)
29. Divide 4x3 + 2x2 + 5x – 6 by 2x2 + 1 + 3x and verify the division algorithm. (2013)
Solution
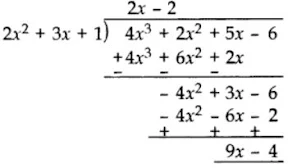
Quotient = 2x – 2
Remainder = 9x – 4
Verification:
Divisor × Quotient + Remainder
= (2x2 + 3x + 1) × (2x – 2) + 9x – 4
= 4x3 – 4x2 + 6x2 – 6x + 2x – 2 + 9x – 4
= 4x3 + 2x2 + 5x – 6
= Dividend
30. If α and β are the zeroes of the polynomial p(x) = 2x2 + 5x + k satisfying the relation, α2 + β2 + αβ = 21/4, then find the value of k.
Solution
We have,
p(x) = 2x2 + 5x + k



Solution
We have, p(x) = kx2 + 4x + 4
Here a = k, b = 4, c = 4

⇒ 24k2 = 16 – 8k
⇒ 24k2 + 8k – 16 = 0
⇒ 3k2 + k – 2 = 0 [Dividing both sides by 8]
⇒ 3k2 + 3k – 2k – 2 = 0
⇒ 3k(k + 1) – 2(k + 1) = 0
⇒ (k + 1)(3k – 2) = 0
⇒ k + 1 = 0 or 3k – 2 = 0
⇒ k = -1 or k = 23

Solution

Remainder = 2x + 3
px + q = 2x + 3
p = 2 and q = 3
36. If α and β are the zeroes the polynomial 2x2 - 4x + 5, find the values of
(i) α2+β2
(ii) 1/α + 1/β
(iii) (α-β)2
(iv) 1/α2 + 1/β2
(v) α2 + β2
Solution
We have,
p(x) = 2x2 - 4x + 5p
If α and β are then zeroes of p(x) = 2x2 - 4x + 5, then

37. If p(x) = x3 – 2x2 + kx + 5 is divided by (x – 2), the remainder is 11. Find k. Hence find all the zeroes of x3 + kx2 + 3x + 1.
Solution
p(x) = x3 – 2x2 + kx + 5,
When x – 2,
p(2) = (2)3 – 2(2)2 + k(2) + 5
⇒ 11 = 8 – 8 + 2k + 5
⇒ 11 – 5 = 2k
⇒ 6 = 2k
⇒ k = 3
Let q(x) = x3 + kx2 + 3x + 1
= x3 + 3x2 + 3x + 1
= x3 + 1 + 3x2 + 3x
= (x)3 + (1)3 + 3x(x + 1)
= (x + 1)3
= (x + 1) (x + 1) (x + 1) [∵ a3 + b3 + 3ab (a + b) = (a + b)3]
All zeroes are:
x + 1 = 0 ⇒ x = -1
x + 1 = 0 ⇒ x = -1
x + 1 = 0 ⇒ x = -1
Hence zeroes are -1, -1 and -1.